percentages Archives | Math for Grownups

In Math for Grownups

Introversion, Extroversion and the Percentage of Personality Type

I am an INTP — introvert, intuitive, thinking, perceiving. If this is all Greek to you, let me be the first to introduce you to the Meyers-Briggs Personality Type. According to the Myers-Briggs Type Indicator (MBTI), this combination of letters means I am conceptual, analytical, intellectually curious, adaptable, independent and critical. I love ideas and pursue understanding.

Sounds exactly like me. Exactly.

I learned my Meyers Briggs personality type by taking a rather involved multiple-choice evaluation. But there are shorter tests online that work reasonably well. If you don’t know your type, check it out here. (I’ll wait.)

It’s pretty trendy to know what your personality type is and to identify the characteristics that you share with others. Being an INTP — remember, I love ideas and pursue understanding — I think this is a really good thing. (Also being an INTP, I think it’s a good idea for folks to get the real Meyers-Briggs test, if they plan to use the results in any serious capacity, like workplace team building or couples therapy.) You don’t have to agree with the veracity of these personality types to find them interesting and entertaining. Personally, I’ve found that knowing my type helps me make decisions — like striking out on my own as a freelance writer.

But what I find in the many, many articles on this subject is how unique the results seem. So many of my friends have said, “Wow! No wonder I feel so [misunderstood/alone/different]! Only 5 percent of the population has the same personality type as I do!”

On some level, and with some things, we all want to feel singular. Seems to me, personality types are one of those things.

But these small percentages have always bugged me a little bit. And that’s because of the math.

There are four preferences in the Meyers-Briggs personality type: introversion vs. extroversion, sensing vs. intuitive, thinking vs. feeling, and judging vs. perceiving. (I won’t get into the details of these characteristics, but do know this: judging isn’t a bad thing at all. Learn more at The Meyers Briggs Foundation website.) Since there are two options per preference, there are 16 possible personality types according to the Meyers-Briggs test.

I think we forget that there are so many different combinations. And that clouds our understanding of what is rare and what is not rare.

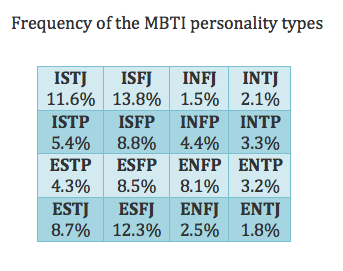
In fact, the Meyers Briggs Foundation has studied the occurrence of each of the 16 personality types in the population.

First, a disclaimer: already, this is not a random sample. The foundation used data that is reported to them, which means that only people who have taken the MBTI evaluation were in the sample studied. But what if people with a certain personality type are less likely to take a personality test? This type would not be accurately represented in the sample. And if one type is more likely to take a personality test? Those folks might appear more often in this sample than in the general population.

Still, let’s take a look.

(Data from the Meyers Briggs Foundation)

If you lined up all of the personality types in order of their percentages, the types at the middle are ISTP (5.4 percent) and INFP (4.4 percent). If you fall within 5 percent of the population, are you unusual? Well, yes. In some regard, but only if the rest of the population falls in one category outside of that 5 percent.

In terms of rarity, we often think of rates of disease. According to the American Autoimmune Related Disease Association, about 5 percent of the population in Europe and North America have an autoimmune disease. With these diseases, (including celiac disease, rheumatoid arthritis, multiple sclerosis, lupus and type I diabetes) the immune system is attacking some part of the body. (In fact, my father had autoimmune hepatitis and vitiligo and died of pulmonary fibrosis.)

If 5 percent of the population is affected by autoimmune disease, 95 percent is not. This makes autoimmune disease seem kind of usual or rare. Actually, it’s not rare by medical standards. For a disease to be considered rare in the U.S., it must affect less than 0.06 percent of the population.

And as with all math, the context matters. There are 16 personality types. If there were only three types, 5 percent is really rare. But with 16 types, well, 5 percent isn’t so unique. That’s because the other 95 percent is spread out among the remaining 15 types.

Now where this stuff gets really interesting is in certain populations. For example, in a 1992 study of college and research librarians, 11.5 percent were INTJ, while 0.8 percent were ESFP. These results definitely don’t square with the frequency in the general population. So you might be not so rare among librarians but more uncommon within the rest of the world.

I don’t mean to suggest that each of us is not a special snowflake. We are — but that’s not because of our personality types. As useful as these categories are, they certainly ignore a large part of the rest of what makes us who we are. (Meyers and Briggs knew this, of course, and their foundation works hard to be sure that the MBTIs are used ethically and responsibly.)

So go on with your special self. Fly your freak flag proudly. Just know that each of the personality types is interesting and unique in its own way. You are special, but not because of your personality type. There are just too many other possibilities!

Photo Credit: db Photography | Demi-Brooke via Compfight cc

Do you know what your MBTI type is? I love to hear about others’ personality types and how they understand them. Share your personality type stories in the comments section. Because you’re special. Just the way you are.

In Personal Finance

4 Math Tricks to Keep You Frugal

If you’ve started down the frugality path, you have probably already been smacked in the face with one unavoidable fact: there’s math involved in living within or below your means. For some, this is no biggie. For others, this could very well be the difference between saving a little and saving a lot.

But even if your basic math skills are rusty, you can handle these calculations, no problem. A few simple tricks will help you stay frugal and even take it up a notch!

Read the rest of the post here.

How has math helped you be frugal? Share your ideas in the comments section here or at Suddenly Frugal!

Would you like me to guest post at your blog? Or do you know of a blog that I would fit right in with? I’ve got lots of ideas to share with anyone who will listen! And I promise I’m a good guest. I wipe out the sink after I brush my teeth and don’t mind if the cat sleeps on my pillow. Get the details here.

In Math for Parents

The Arithmetic of Allowance

You probably find it pretty darned easy to encourage literacy. In fact, there are countless magazine articles and books and workshops out there on this very subject. And so all good parents read to their kids every night, play word games with them, give them magnetic letters for the fridge.

But what about math? If you’re like most parents, the idea of working math into the day probably seems down right daunting. Scary even.

It’s not as hard as you think, especially if you’re willing to give into your children’s demands for a regular allowance. Money is an instant math lesson—and can motivate even the most reluctant student (adult or child).

Want to know how? Read my guest post on Simply Budgeted.

Would you like me to guest post at your blog? Or do you know of a blog that I would fit right in with? I’ve got lots of ideas to share with anyone who will listen! And I promise I’m a good guest. I wipe out the sink after I brush my teeth and don’t mind if the cat sleeps on my pillow. Get the details here.

In Math at Work Monday

Math at Work Monday: Kim the Copywriter

If you’ve ever visited the website of a prescription medication or picked up a brochure from your doctor’s office, you’ve seen the kind of work that Kim Hooper does. And she’s proof that math and writing are not mutually exclusive endeavors.

As a senior copywriter for an advertising agency, Kim writes brochures, websites and other copy that helps promote a brand or a product. Since her agency’s primary client is a pharmaceutical company, much of her writing is science-based.

When do you use basic math in your job?

Much of my job involves scanning through research papers about specific drugs and interpreting clinical data in a “sexy,” Madison Avenue way. This tends to involve a bit of math. For example, let’s say we want to point out that our drug is really successful with women over 40 years old. I will look through the demographic tables in the clinical study to create a compelling factoid. Let’s also say that out of 100 women, 60 are over 40 years old. So, when writing a piece, I may have a big headline that says something like, “60% of women in the clinical study were over 40 years old.”

Most of the math I do involves basic addition or subtraction and percentage calculations. Very often, I’ll do percentage calculations for side-effects data. So if 3 patients out of 150 in the clinical study experienced side effects, I’ll take this fact and make sure to call out that 98% of patients did not experience side effects.

Do you use any technology (like calculators or computers) to help with this math?

I do use the calculator built into my PC to double check my work. But I almost always have to do “margin math,” meaning I show my calculations on paper so the client’s regulatory committee can review them.

How do you think math helps you do your job better?

Math keeps my left brain strong. In advertising, the right brain is very important. This is a creative business. We’re trying to find interesting, compelling ways to communicate product messages that may not be that thrilling at first glance. My left brain can help make the messages thrilling. Numbers are very appealing to consumers. If they can see information broken down into easy-to-understand percentages, for example, they may be more likely to try our medication over another one.

How comfortable are you with math?

I’ve always been a bit of a math nerd, and I went all the way through Advanced Placement Calculus in high school. In fact, it was really difficult for me to choose a major in college because I loved math and science and I also loved the arts. For a short time, I double-majored in genetics and psychology. I ended up majoring in communications, which seemed broad enough for me to explore a number of career options. I just happened to fall into a career that makes use of both sides of my brain, which I love. I really enjoy sifting through data and doing the math necessary to make facts come to life.

I think we all get a little rusty if we don’t use math regularly, but it’s been part of my job for a number of years now. There’s no way I could do calculus again, but I have no problem doing basic math. I enjoy it.

Kim Hooper is an advertising copywriter by day, novelist by night. Get to know her work at KimHooperWrites.com.

Do you have questions for Kim? If so, ask them in the comments section!

In Math at Work Monday

Math at Work Monday: Beth the registered nurse

Beth Hanes is a registered nurse in a plastic surgery center. She takes care of patients before, during and after their surgeries. Here’s how she uses math everyday.

What kind of math do you use in your job?

I use basic math for a lot of things, but probably the most important calculations are the ones related to medication use. Sometimes I dilute medication before giving it. For example, Promethazine needs to be diluted before it’s given in an IV. Using a 10mL syringe, I draw up 1mL of Promethazine and then add 9mL of normal saline (0.9% sodium chloride) to create a 10% Promethazine solution.

I also use basic math to determine, based on body weight, how much medication to administer. Medications are generally given on a milligram per kilogram basis. So, I convert a person’s weight in pounds to weight in kilograms (divide pounds by 2.2 to obtain kilograms), then I multiply this number of kilograms by the number of milligrams per kilogram to get the correct dosage. For example, Lidocaine might be ordered as 1mg/kg. A 220-pound patient weighs 100kg, so the correct dosage is be 100mg of Lidocaine.

How do you do your calculations?

I do use calculators because they’re typically faster, but I think it’s important to know how to do math by hand. I usually don’t have a calculator on hand in the operating room! Also, it’s critically important for me to have basic formulas memorized (such as how to convert pounds to kilograms). Without that knowledge, having a calculator or not is irrelevant.

Why is math important for your job?

Math skills help me ensure patient safety. There was a highly publicized case a few years ago in which actor Dennis Quaid’s infant twins were administered a very high dose of Heparin. This error occurred for many reasons, but one key factor was doing the math involved. This is a classic case of calculating dosage based on weight, and obviously errors were made in that calculation. In nursing, if you misplace a decimal point, you can kill someone.

When it comes to math in nursing, I think the main thing is to be very careful about calculations, double-check them, and then have someone else double-check them. No matter how good you may be at math, anyone can misplace a decimal point when calculating on-the-fly. It’s much better to take the extra seconds to have someone review your calculations and keep patients safe than to have any sense of ego about your math ability and endanger a patient.

What kind of math did you take in high school?

I had a rather sketchy math education, because my parents moved around a lot, and I only made it through Algebra II. On the other hand, advanced math was not yet common at the high school level when I was that age. Calculus, for example, was a college course. I did not feel I was good at math in high school. However, this “low math esteem” led me to focus on practicing real-world math skills.

These days, I am fairly comfortable with math, in general, though I frequently have to think through conversion problems, which are common in nursing. I find I often want to divide when I should multiply, for instance, so I have to be careful about that! Once I have a formula memorized, however, I feel very comfortable substituting variables with real values and arriving at the correct answer.

If you have questions for Beth, ask them in the comments section. Oh, and today, June 20, is her birthday! So take minute to wish her a happy day!

Read other Math at Work Monday entries in the archive. And if you or someone you know wants to be interviewed for this regular, Monday feature, let me know.

In Math at Work Monday

Math at Work Monday: Melissa the Speech Therapist

When you were in middle and high school, did you think you’d never need to use the math you were learning? Like most grownups, you probably found out that, yes, you did need some of it — and some of it you’ll never do again.

Each Monday, I’ll introduce you to someone who uses math on the job. We’re going to skip over the engineers, physicists and statisticians and stick to folks who use regular, everyday math. First up, my sister, Melissa, a speech therapist.

So, what kind of speech therapist are you, and how long have you been doing this job?

Lots of people think of speech therapists in the school setting, working with kids. But I work with adults, who are critically ill or recovering from an injury or illness in rehab. I’ve been doing this for 19 years. Basically, I provide care in these areas:

Speech and communication: patients who have declined neurologically or physically and cannot talk, have slurred speech, or any difficulty communicating.
Swallowing: patients who have swallowing impairments, usually from neurological deficits, or physical decline in some way.
Cognitive: patients whose thinking skills have declined, including memory, problem solving, attention, reasoning.
Other specialty areas: patients with tracheotomies, laryngectomies, concussions.

All of my patients are adults, and most of them have had some sort of illness or injury.

When do you use math in your job?

Rehab is very goal oriented. I meet with patients on a regular basis and keep data on those goals — how many questions the patient answered correctly or the number of times a he performed a certain task properly. At the end of the day, I tabulate that data. And then at the end of the week, I average the data for the week.

I also use math in some of my tasks with the patients.
Functional math is a form of reasoning, and so I will provide a patient
with math tasks to “rehab” his or her reasoning skills.

These calculations help me determine if the patient has met that goal, and if so, I create a new goal.

I also use math in some of my tasks with the patients. Functional math is a form of reasoning, and so I will provide a patient with math tasks to “rehab” his or her reasoning skills.

What kind of math is important for your job?

Percents! If the patient got 8 out of 10 correct on a certain task, I put in the note that she was 80% successful. (But sometimes the numbers aren’t great for mental math: 29 out of 44 correct, for example.)

Most rehab and acute care settings use a very specific form of measuring assistance, called Functional Independent Measures, or FIMS.

Independent: 100%
Modified Indpendent: 100% with extra time
Supervision: 90% or above
Minimum Assist: 75-90%
Moderate Assist: 50-75%
Maximum Assist: 25-50%
Dependent: less than 25%

So, since I measure FIMS weekly, I am always creating percentages in my head (and on paper) of how a patient is performing on that certain task.

How does math help you do your job?

It allows me to be very accurate in data collection. Of course, many patients’ lengths of stay is dependent on whether or not there is proven progress, and the best way to prove it is to show it in black-and-white. Patients and their families would rather see “80% accuracy” as opposed to “required min assist.” Percents are more accurate and detailed.

I also firmly believe that having patients do math themselves helps them building their reasoning skills. I think I am doing my job better by making them do math! I will even have my patients average their data for the week. This helps them use reasoning skills as well as understand their goals and how they are progressing.

How comfortable with math do you feel?

I feel comfortable with simple math, especially if I can write it out, use pen and paper, etc. I get bogged down with more complex actions and definitions, but I don’t have to use these in my job.

Did you have to learn to use new skills?

No, I use basic elementary and middle school math. However, I do feel like it took me years in my job to realize that I could involve the patient to help me! It’s a great way to help the patient therapeutically. I think I forget to make math functional.

Thank you, Melissa for being the first person featured in Math at Work Monday! If you have questions for Melissa, feel free to ask them in the comments section. And if you know of someone who uses regular math in their jobs — duh, of course you do! — and you would like to see that person featured here, drop me a line and let me know!

In Personal Finance

Is Your Boss Ripping You Off?

In last Friday’s Open Thread discussion, Gretchen posted this question:

My husband’s company does not provide health insurance for me and the kids, which is a $12,000 value. In his field, there is a salary scale based on education, number of years experience, geography, etc. The salary scale assumes that the employer provides health insurance for the family. His salary is currently at 79% of the scale, and his employer wants to eventually get him up to 100%. But that doesn’t include the insurance, so it won’t really be at 100% and is not now really at 79%. But I can’t figure out which way to do the math so he can show them the actual percentage. They’re saying he’s at 79 percent. I’m saying it’s lower because they aren’t accounting for that $12K.

All of that boils down to this: What percent of the salary scale is Gretchen’s husband actually making, given that he, and not his employer, pays the $12,000 bill for insurance? There are two steps to this problem:

1. Find the actual salary that is at 100% of the scale.

2. Find the actual percent of Gretchen’s husband’s salary, minus the cost of insurance.

I’m going to tell you up front that we’re going to use a proportion here. What is proportions? A proportion is two equal ratios. So, if you have two fractions with an equal sign between them, you have a proportion.

And how did I know to use a proportion? Well, my big clue was that we’re working with percents. Percent means “per one hundred,” and per one hundred means “out of one hundred,” which just means, “put the percent value over 100.” In other words:

79% = 79/100

The tricky part is figuring out what the proportions should be.

Step 1:

salary/x = 79/100,

where “salary” is Gretchen’s husband’s salary, and x is the top salary on the scale.

That’s because the company assumes that your husband’s salary is 79% of the scale. (Notice this: “salary” and “79″ are in the numerators — or top values of the fractions.)

To solve this proportion, we need to plug in Gretchen’s husband’s salary and then solve for x. In order to make this easy to explain, I’m going to assume that his salary is $100,000.

substitute: {$100,000}/x = 79/100 cross multiply: {$100,000*100} = 79x simplify: {$10,000,000} = 79x solve for x: $126,582 = x

So if his salary is $100,000, the top salary on the scale is $126,582.

Step 2:

{$100,000-12,000}/{126,582} = p/100,

where p is the actual percent of the scale.

Let’s look carefully at this proportion: The first ratio is just the salary minus the cost of insurance, over the max salary in the scale. (That’s what we found in step 1.) The second ratio is just like the second ratio in step 1, except that we don’t know what the percent is.

Now, pay close attention to this. Check the top numbers to be sure they match. We want to know the actual percent of the scale that Gretchen’s husband is making — and that’s what’s represented in the top number of each ration.

Check the bottom numbers to be sure they match. Do they? Why yes! Yes they do! That’s because $126,582 is 100% of the salary scale.

(Unlike my 10-year-old daughter’s outfits, math is very matchy-matchy. Knowing that will help you organize your problems and check to see if they’re set up properly.)

Now all we need to do is solve for p.

simplify: {$88,000}/{126,582} = p/100 cross multiply: {$88,000*100} = {126,582p} simplify: {$8,800,000} = {126,582p} solve for p: 69.5 = p

So what does this mean? If Gretchen’s husband makes $100,000 a year and is paying $12,000 for insurance, he’s earning 69.6% of the salary scale.

If you made it this far, you get a gold star! Pat yourself on the back, and take the rest of the day off. This is a complex problem that depends on an understanding of proportions and how to solve for a variable in an algebraic equation.

Never fear! I’ll unravel some of these mysteries in later blog posts. And of course, if you have a question, ask it in the comments section!